Cmuse.org recently contemplated the “Golden Ratio” and Fibonacci Sequence and looked at some of their applications in Classical music composition. The site says:
“During the fifth century BC, the Greeks determined that there was a proportion that recurred in geometry, nature and architecture, that embodied the quintessential concept of harmony. The Parthenon was built according to that principle.
“Two centuries later, Euclid provided us with the first definition of that proportion, explaining to us how to calculate what is now commonly known as “the golden ratio”.
“A straight line is said to have been cut in extreme and mean ratio when, as the whole line is to the greater segment, so is the greater to the lesser.” The result is an irrational number that roughly corresponds to 1.618 and, expressed by a decimal fraction, the golden ratio amounts to 0.618. The formula can be written as x/(1-x)= (1-x)/1.
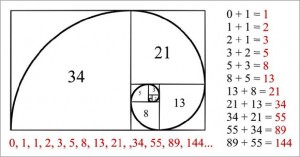
“In the thirteenth century, Italian mathematician Leonardo Bonacci (also known as Fibonacci) posed and solved a problem regarding the growth of rabbit population, by illustrating a series of numbers where each number is the sum of the previous two numbers. Even though Fibonacci did not observe it in his calculations, the limit of the ratio of consecutive numbers in this sequence nears 1.618, namely the golden ratio.
“Both the golden ratio and fibonacci sequences abound in art, nature and geometry. So what? you may ask now. If, by now, you’re wondering what all these numbers (I was really weak in mathematics) have to do with music, listen to the following five compositions, which allegedly contain references to the golden ratio and/or the Fibonacci sequence. In fact, there must have been a good reason for Antonin Dvorak to say ‘Mozart is sunshine.’”
The site then discusses pieces by Mozart, Beethoven, Bartok and others and includes audio
http://www.cmuse.org/classical-pieces-with-the-golden-ratio/
thanks for Soror Hypatia for the tip!